
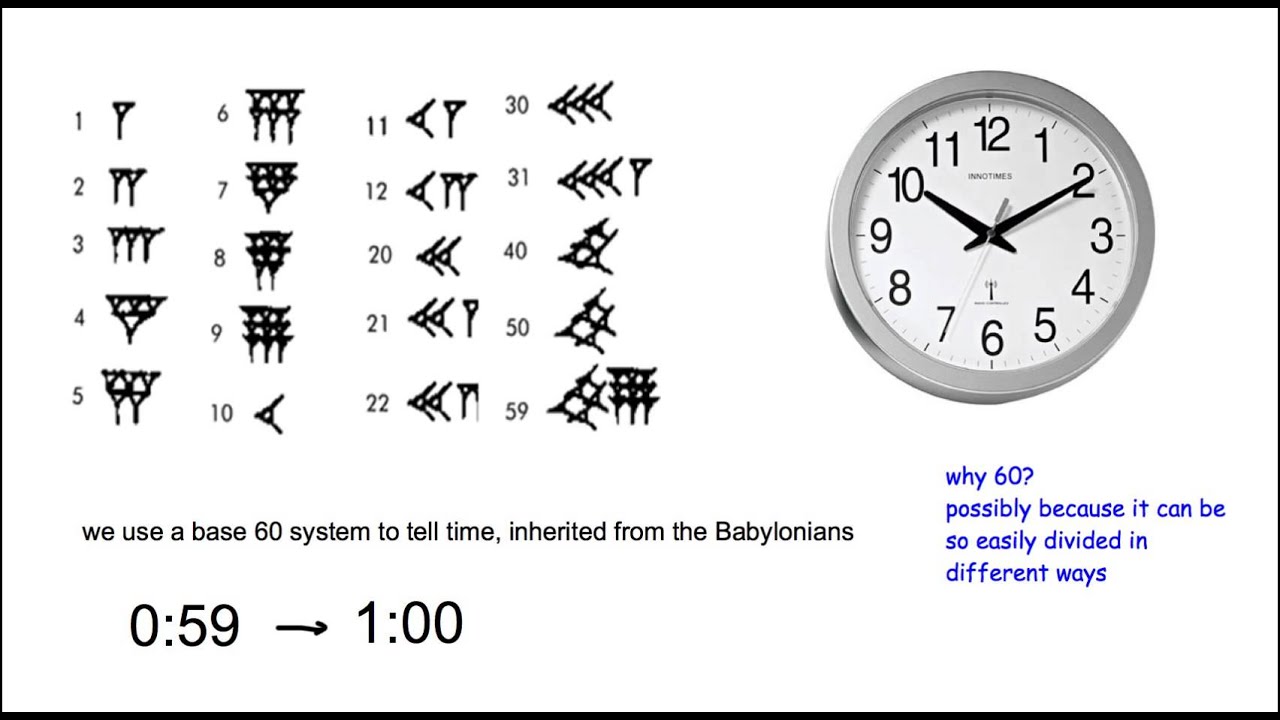

It also had buildings that towered 110 feet above the jungle floor (Bazin 2002). By the third century, one city, Palenque had its own modern drainage system and observatories (Bazin, 2002). They built large temples covered in stucco, many still standing today. They studied the cycles of the moon, earth, and other planets (Bazin, 2002). The Mayas built roads to connect their cities and built structures that are still considered amazing today (Bazin, 2002). The first sign of their being in this area was around 2600 BCE. The Mayas prospered in an area ranging from southern Mexico and the Yucatán Peninsula through Belize, Guatemala, Honduras, and El Salvador (Bazin, 2002). Rather than counting fingers, the Egyptians counted the joints on each of the four fingers on a hand and there are three joints per finger giving twelve joints on a hand. The Babylonians also came up with the concept of a degree being divided into 60 minutes with each minute being divided into 60 seconds. The Babylonians came up with the concept of dividing a day into 24 hours with each hour having 60 minutes and each minute 60 seconds. The number 60 was significant to the Babylonians. It is believed that the Mayans used base 20 because they lived in a warm climate where they did not wear shoes, thus giving 20 fingers and toes. The Mayans used a vigesimal (base 20) number system, the Babylonians used a sexagesimal (base 60) number system, and the Egyptians used a duo-decimal (base 12) number system. In base 10, ten digits are used and those digits are 0 through 9. We use base 10 because we have 10 fingers. History of Bases Used in Ancient Civilizations (previous) .EDUC 525 - The Converter Box - Number Systems 2014: Christopher Clapham and James Nicholson: The Concise Oxford Dictionary of Mathematics (5th ed.) .(next): Chapter $1$: Tokens, Tallies and Tablets: The first numerals 2008: Ian Stewart: Taming the Infinite .The number represented in the Babylonian number system as: The number $25 \, 267$ is represented in the Babylonian number system as: In such a system, the radix point is represented by a semicolon. Hence it is commonplace to use their decimal counterparts, separated by commas, so that the number represented, for example, in cuneiform as: When representing numbers using the Babylonian number system, it is laborious to represent the actual cuneiform symbols themselves.
#Using the babylonian numerals. full#
The fact that they had no symbol to indicate the zero digit means that this was not a true positional numeral system as such.įor informal everyday arithmetic, they used a decimal system which was the decimal part of the full sexagesimal system. Instead, the distinction was inferred by context. The rightmost grouping would indicate a number from $1$ to $59$ the one to the left of that would indicate a number from $60 \times 1$ to $60 \times 59$Īnd so on, each grouping further to the left indicating another multiplication by $60$įor fractional numbers there was no actual radix point. Thus these groupings were placed side by side: The characters were written in cuneiform by a combination of:Ī thin vertical wedge shape, to indicate the digit $1$ a fat horizontal wedge shape, to indicate the digit $10$Īrranged in groups to indicate the digits $2$ to $9$ and $20$ to $50$.Īt $59$ the pattern stops, and the number $60$ is represented by the digit $1$ once again. The number system as used in the Old Babylonian empire was a positional numeral system where the number base was a combination of decimal ( base $10$) and sexagesimal ( base $60$).


 0 kommentar(er)
0 kommentar(er)
